
(This follows from Niven's theorem.) They are most useful in that they may be easily remembered and any multiple of the sides produces the same relationship. Right triangles whose sides are of integer lengths, with the sides collectively known as Pythagorean triples, possess angles that cannot all be rational numbers of degrees. The right angle is 90°, leaving the remaining angle to be 30°. After dividing by 3, the angle α + δ must be 60°. The proof of this fact is simple and follows on from the fact that if α, α + δ, α + 2 δ are the angles in the progression then the sum of the angles 3 α + 3 δ = 180°.
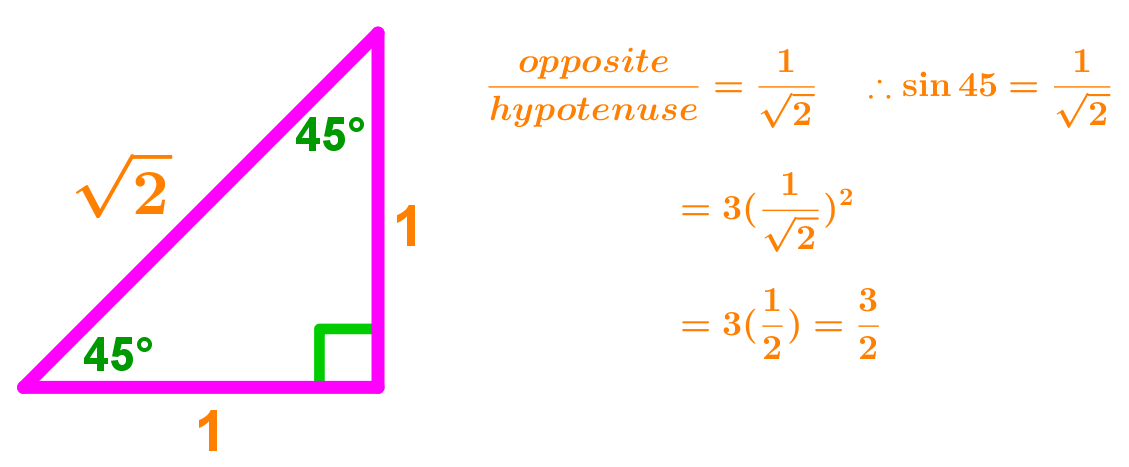
The 30°–60°–90° triangle is the only right triangle whose angles are in an arithmetic progression. The fact that the remaining leg AD has length √ 3 follows immediately from the Pythagorean theorem. Then ABD is a 30°–60°–90° triangle with hypotenuse of length 2, and base BD of length 1. The geometric proof is:ĭraw an equilateral triangle ABC with side length 2 and with point D as the midpoint of segment BC. The proof of this fact is clear using trigonometry. This is a triangle whose three angles are in the ratio 1 : 2 : 3 and respectively measure 30° ( π / 6), 60° ( π / 3), and 90° ( π / 2). The side lengths of a 30°–60°–90° triangle Special triangles are used to aid in calculating common trigonometric functions, as below: This approach may be used to rapidly reproduce the values of trigonometric functions for the angles 30°, 45°, and 60°. The side lengths are generally deduced from the basis of the unit circle or other geometric methods. The angles of these triangles are such that the larger (right) angle, which is 90 degrees or π / 2 radians, is equal to the sum of the other two angles. "Angle-based" special right triangles are specified by the relationships of the angles of which the triangle is composed. Special angle-based triangles inscribed in a unit circle are handy for visualizing and remembering trigonometric functions of multiples of 30 and 45 degrees.


 0 kommentar(er)
0 kommentar(er)
